PHOTONICS APPLIED: FREQUENCY COMBS: Optical frequency combs forge precise optical clocks and more

Besides applications in metrology (most notably, optical clocks), highly stable optical frequency combs are playing a role in ultraprecise spectroscopy for astronomy and in other emerging areas. Frequency combs became a hot research topic in 1999 and even more so after the Nobel Prize was awarded to John L. Hall and Theodor W. Hänsch in 2005 for their work in this area. Since then, frequency combs have found their way into various areas of research and technology—most notably for optical clock applications.

It has been known for decades that the optical spectrum of the output of a modelocked laser, generating a train of picosecond or femtosecond light pulses, consists of discrete lines. Such a line structure is called a frequency comb. For comparison, continuous-wave lasers also often generate a spectrum consisting of discrete lines; however, only in modelocked lasers are the frequencies of these lines tightly synchronized with each other, despite influences of chromatic dispersion and optical nonlinearities.

In an idealized picture without any noise, all line frequencies are exactly determined by the simple formula ν_j = ν_ceo + j f_rep, wherejis an integer index,ν_ceois the so-called carrier-envelope offset frequency, andf_repis the pulse repetition rate. In reality, there are some noise influences leading to a finite frequency width of the lines and to drifts of the parametersν_ceoandf_rep. However, such noise influences can be kept rather low with a combination of measures: choosing suitable operation parameters (such as pulse energy and laser gain), making a very stable laser setup, carefully shielding the setup against external influences such as temperature fluctuations and pump power fluctuations, and in many (but not all) cases stabilizing ν_ceoand/orf_repto external references (such as a continuous-wave optical frequency standard or a microwave oscillator) using common control techniques. With such methods, the frequencies involved and the relationship between them can be made exceptionally stable.

Initially, titanium:sapphire (Ti:sapphire) lasers had a dominant role in frequency comb generation. In recent years, however, a number of more practical systems have been developed, including various rare-earth-doped laser systems (that can be directly diode-pumped), being partially fiber-laser-based systems. Whereas the performance of Ti:sapphire is still usually the best, such alternative systems allow frequency combs to be used in a wider range of applications, including those that are more cost-sensitive.

Frequency combs as optical rulers

A conceptually simple but powerful application of a precise frequency comb source is its use as an optical ruler in high-precision spectroscopy. Astronomy observations, for example, often involve the use of high-resolution spectrometers for analyzing the spectral content of the collected light. In cases where extreme spectral precision is required—like in the search for exoplanets—calibration of the spectrometer can be very demanding. This is particularly true when a huge spectral range is analyzed: Even if several calibration sources in the form of ultraprecise continuous-wave optical frequency standards are used, there are huge spectral gaps where precise calibration remains difficult.

Frequency combs provide a convenient solution to this problem. One can simply inject the light from a stabilized frequency comb source into the spectrometer. The spectrometer output then displays a huge number of well-defined lines, the frequencies of which are known with extremely high precision. This makes it easy to determine with similar precision the frequencies of any astronomical signals (see Fig. 1).

For such applications, it is often desirable that the frequency comb covers a very broad spectral range. Also, the repetition rate should be high enough (10 GHz or even higher) that the line spacing can be well resolved by the spectrometer. The challenge in developing such frequency comb sources lies in achieving the combination of broad spectral coverage in the spectral regions of interest and high pulse repetition rate. Note that spectral broadening using fiber nonlinearities, for example, is not particularly easy in this domain, where the pulse energy is usually quite low due to the high pulse repetition rate. Ti:sapphire lasers with very compact resonators constitute a solution for very broad spectral coverage—although not far into the visible spectral region—and reasonable pulse energies. In some cases, lower pulse repetition rates are used, and the spectrum is filtered afterwards to obtain the desired frequency spacing.

Generating precise microwave clock signals

Cesium atomic clocks have been used as ultraprecise frequency standards for many years. However, optical frequency standards will be preferable in the long run. The main reason is that optical frequency standards based on laser-cooled ions or atoms are already surpassing any microwave-based standards in terms of long-term frequency stability. Another reason is that the high optical frequencies facilitate rapid frequency comparisons: Within a short time, two optical signals can be compared over many oscillation cycles.

For a long time, the challenge of using optical frequency standards was simply that no convenient method was known to phase-coherently translate their output into microwave (or lower-frequency) signals. In other words, a suitable clockwork was not available. So-called frequency chains were realized in a few laboratories, but they were extremely complicated and not practical outside a few specialized labs. Also, it was difficult to compare different optical frequency standards operating in different spectral regions.

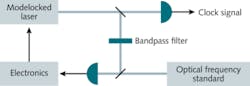
Frequency combs solve these problems. As a simple example, a frequency comb source may be stabilized by locking one line to a highly precise optical frequency standard (see Fig. 2). If the carrier-envelope offset frequency is also stabilized, the pulse repetition rate is directly related to that frequency and to the optical standard, if only the integer index of the stabilized line is known (that index can be determined without requiring uttermost measurement precision). Of course, the repetition frequency can be obtained as an electronic signal simply by sending the pulse train to a fast photodiode.

FIGURE 2. In a simple setup, one line of a frequency comb is locked to an optical frequency standard using a beat signal on a photodetector. The carrier-envelope offset frequency is also stabilized (with components that are not shown here). Another photodetector provides the clock signal.

Time signals can, of course, be distributed with electrical cables. However, the distribution in optical form is often more convenient. Optical signals can simply be transmitted through optical fibers, which are much thinner and cheaper than microwave cables, while exhibiting much lower transmission losses. For these reasons, optical time signals can easily be transmitted over many kilometers. Noise influences in optical fibers due to temperature drifts or vibrations can be substantial, but they can be largely compensated with certain techniques such as detecting additional backward-traveling pulses.

Comparing optical frequency standards

Frequency combs can also be used for comparing the frequencies of different optical frequency standards—even if these operate in quite different spectral regions so that a simple beat-frequency measurement is not possible. A broadband frequency comb may cover a full octave or even more, such that a beat note can be recorded with each frequency standard. It is then possible to obtain the frequency difference between the two standards by taking some integer multiple of the repetition frequency and adding or subtracting the two beat frequencies (see Fig. 3).

FIGURE 3. A setup shows how to compare two optical frequencies that may be in different spectral regions but are both covered by the frequency comb from the modelocked laser.

Even if a frequency comb may not directly cover the full spectral region of interest, further spectral expansion is relatively simple to achieve using nonlinear optical effects. Particularly for pulse repetition rates that are not too high, the pulse energies are sufficient to effectively drive nonlinear effects even in short pieces of glass fiber. For comparison, the power level of single-frequency sources is often too low for efficient nonlinear conversion processes; also, such processes could only allow access to a few additional optical frequencies. The nonlinear spectral broadening process can also introduce additional noise, but that effect is usually not too strong if the initial pulse duration is sufficiently short.

Is comb stabilization mandatory?

Conceptually, most applications of frequency combs are simplest when frequency-stabilized combs are used. In many situations, however, it is better to accept some substantial noise level in a frequency comb and just record the fluctuations, rather than suppressing them by means of feedback control. The main problem is that high-frequency noise influences are hard to suppress, because such control loops have a limited control bandwidth. Also, as the line frequencies are determined by two parameters—the carrier-envelope frequency and the pulse repetition rate—two different control parameters are needed. Most of these parameters tend to influence both frequencies, and typically with different control bandwidths, making it difficult to obtain orthogonal responses. For such reasons, it is challenging to effectively suppress any noise in both frequency parameters.

Such problems are avoided when the frequency fluctuations are only recorded in order to compensate them in the data processing step—possibly with software. Noise compensation is then possible with simpler hardware and in a substantially larger bandwidth, since the measurement bandwidth is often easier to maximize than the control bandwidth.

Problems can arise when the carrier-envelope frequency approaches zero. Therefore, it may be necessary to roughly stabilize the carrier-envelope frequency, preventing it from getting close to zero at any time. This kind of single-parameter stabilization, however, is often much simpler than a full-blown comb stabilization with maximized control bandwidth.