Optical Computing: Optics do not always compute

Optical computing—via photons instead of electrons—has long appealed to researchers as a way of overcoming the limitations of electronics and achieving ultrahigh performance. Photons, for example, travel faster than electrons and do not radiate energy even at high frequencies. Light beams also can pass through each other, allowing high degrees of parallelism. Nevertheless, the practical realization of optical computing always has seemed to retreat over the horizon, while electronic computers became faster, smaller, and cheaper.

Today, most optical-computing scientists are exploring new directions and are no longer seeking to exactly imitate the function of electronic computers. Instead, the focus is on utilizing the inherent strengths of optical devices. It will be many years before these new approaches produce general computers, if they ever do. In the meantime, specialized optical computers, such as optical correlators, are slowly beginning to penetrate commercial markets—potentially driving the wedge needed to expand interest and funding in the field.

Correlators for the market

Currently, optical correlators are the only types of optical computers available on a commercial basis. These devices use an optical processing technique to identify and locate objects of a given shape, a task that is useful in artificial vision, inspection, robotics, and optical character recognition.

Correlators rely on the fact that passing light from an image through a lens performs a Fourier transform. That is, the distribution in space of the light beam is a representation of the image in the frequency domain. Here, frequency refers to the image itself—how much of the image can be represented in terms of large spatial waves and how much in terms of short spatial waves. An image of hair or grass, for example, would have a large high-frequency component, while an image of rolling hills would have a large low-frequency component.

In a correlator, collimated light from a laser passes though a liquid-crystal display (LCD) that contains the image and is then focused by a lens onto another LCD array, which contains the filter. The filter is computer-generated as a Fourier transform of the object to be identified—say, a metal part. The waveform from the image and the filter form an interference pattern that contains a high peak of light at the location of the object in the image (see Fig. 1).

While the optical correlator has been in development for years, it has remained mostly a laboratory device requiring careful optical alignment and custom-designed hardware and software. Recently, though, the National Optics Institute (Quebec City, Canada) began marketing a correlator that works as a plug-in black box interfacing with standard VGA-format cameras and personal computers.¹ The correlator provides real-time performance, allowing an object to be tracked frame by frame via an image from a video camera.

Because the correlator uses interference patterns to recognize shapes, the signal intensity just rolls off gracefully as the orientation of the object changes relative to that in the filter. "We can recognize objects that have rotated 45° and, by detecting the intensity of the signal, measure the orientation as well," said Alain Bergeron, who led the Advanced Imaging Systems group in developing the correlator. The compact size, standard components, and relatively modest price of the correlator could make an artificial vision system much more powerful and commonplace in a few years.

Optical computers are distant providers

While optical correlators are moving into the market place, a general-purpose optical computer remains elusive. The use of free-space lasers—which opens up the possibility of high degrees of parallelism—faces several daunting challenges. In such an approach, spatial light modulators encode data on an array of light beams. Problems arise when this light must interact with other light to produce results. Amplification is generally low or nonexistent, and alignment and miniaturization of free-space devices can be extremely difficult.

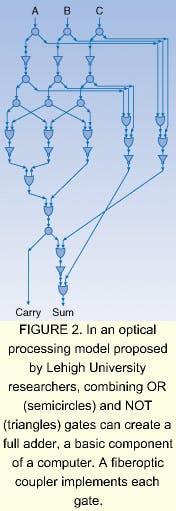
Several possible solutions to these problems have been reported, but all are still in the early stages of research. One approach is to abandon the highly parallel advantages of free-space arrays for the greater speed and control of fiberoptic-based computation. Researcher Alastair McAulay at Lehigh University (Bethlehem, PA) has developed a design based on fiberoptic couplers as the basic elements to create NOT and OR logical gates from which can be built any arithmetic or logical operation.²

According to McAulay, all signals are produced by synchronized, polarized laser diodes. By shifting the phases of the signals, they can be made to interfere constructively or destructively in the couplers to generate the logical functions. The amplification needed to keep the process going is provided by semiconductor optical amplifiers, which are closely related to laser diodes. As all operations take place optically without any conversion back to electronic form (except perhaps for input and output), gigabit-per-second throughputs are easy to achieve. By combining 30 of the elementary gates, McAulay has designed full adders, which are the basis for all other arithmetic operations (see Fig. 2). So far, though, he has yet to obtain the funding for experimental tests of his ideas.

Order out of chaos

A more-radical approach is to abandon a purely digital model of computation and instead exploit some of the inherent phenomena of optical systems. At the Georgia Institute of Technology Applied Chaos Laboratory (Atlanta, GA), William Ditto, working with Sedeshna Sinha of the Institute of Mathematical Sciences (Madras, India), is investigating the use of ultrafast chaotic lasers for computation.³ Their reasoning is that because chaotic systems seem to be common in living organisms, including the human brain, they probably evolved for use in processing information.

In certain conditions, lasers oscillate in a chaotic manner that is not actually random, but rather governed by laws that naturally generate complex patterns very sensitive to small changes in the system. By linking a simple network of computer-simulated chaotic laser oscillators together, Ditto and Sinha were able to control the operations. They used an adaptive connecting link that would open whenever the level of oscillation in a given element exceeded a threshold value.

The researchers then encoded numbers in the behavior of the chaotic lattice using a variety of techniques and either designating certain patterns of oscillation as certain numbers or relating numbers to the amplitude or frequency of oscillations. The lattice was then allowed to naturally interact with itself to produce an "output" that encoded the results of the chaotic "calculation."

Altering the connections allowed altering the type of calculation performed. Although the detailed behavior of the lattice was unpredictable, the team could "program" it to produce a reliably correct answer. Because the oscillations were at optical frequencies, calculations could be performed at extremely high rates.

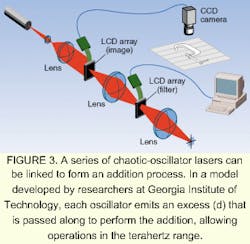
In one simulation of this technique, the excess oscillations of each chaotic element are conveyed through the threshold connection to another element, triggering an avalanche toward the output. Adjusting the thresholds allows the oscillators to perform simple additions (see Fig. 3). While this task may not seem impressive, it will be if it can be accomplished with real laser oscillators that can oscillate at terahertz rates.

Again, even this work remains theoretical, because the team used simulated lasers. Still, Ditto hopes to soon implement the system experimentally. If his work is successful, he believes that such chaotic computers could perform arithmetic pattern detection and Fourier transforms faster than conventional electronic computers.