Terawatt lasers produce faster electron acceleration
DONALD UMSTADTER
Recent technological developments in the design of high-peak-power lasers and novel ideas about how to use them to accelerate electrons are about to revolutionize accelerators and high-energy photon sources. Ever since the development of the chirped pulse amplification (CPA) technique in 1987, the size of high power lasers has been decreasing.1 Table-top-size lasers can now produce peak powers in the range of tens of terawatts and can be focused to produce the highest electromagnetic intensities ever achieved, exceeding 1020 W/cm2. Linear accelerators, however, in terms of field gradient, have not changed much since they were first conceived and built; in order to achieve greater acceleration, their length must be increased correspondingly. This is because dielectric breakdown of the radio-frequency electric fields on the cavity walls limits the maximum field gradients to less than or equal to 1 MV/cm. Lasers, on the other hand, can be used to accelerate electrons via the electrostatic fields of large-amplitude plasma waves,2 which, because breakdown cannot occur, have a maximum axial electric field predicted to be three orders of magnitude higher (2.5 GV/cm).
Consequently, just as the size of high-power lasers has recently been reduced by several orders of magnitude, a similar reduction may soon occur in the size of accelerators and the high-energy photon sources that use them. One can imagine accelerating electrons with this technique over a distance of just a few meters to the same final energy as is obtained after a distance of two miles with the Stanford linear accelerator (SLAC), currently the world's largest. Moreover, laser accelerators may also generate ultrashort-pulse (femtosecond) electron bunches, which are absolutely synchronized to an ultrashort-pulse laser and thus are uniquely suited for the study of ultrafast dynamics in physics, chemistry, and biology.
The field at the focus of one of these short-pulse, high-power lasers is so high that electrons oscillate at nearly the speed of light, giving rise to several interesting, and previously unstudied, effects. For instance, it produces extremely high laser pressure (the ponderomotive force), which can drive a high-amplitude plasma or wake-field plasma wave, the basis for what is called the laser wake-field accelerator (LWFA). Essentially, the laser pulse pushes the electrons out of its way, but the ions—because of their much larger mass—pull them back, setting up a plasma wave oscillation in its wake. In this way, the plasma wave effectively rectifies the laser electromagnetic field so that it becomes an electrostatic field propagating in the direction of the light pulse at nearly the speed of light. This process can continuously accelerate electrons to gigaelectronvolt (GeV) energies in a centimeter distance.
Normally, light will diffract in a distance equal to a Rayleigh range, which, in order to reach high peak intensities, can be quite short. In order to achieve the intensities required to reach GeV energies with current table-top lasers, some form of light guiding must be used. Fortunately, several means are available to accomplish this. Besides producing a high-amplitude plasma wave, the high velocity motion of the quivering electrons can relativistically self-focus the laser beam. This arises from the mass change of the plasma electrons as they quiver in the laser field, which in turn changes the plasma frequency.
Because the intensity is radially dependent, so then is the plasma refractive index, which acts as a positive lens. Self-focusing also creates a self-guided channel, significantly increasing the distance over which the light would normally propagate (and thus over which the plasma wave can grow and the electrons can be accelerated). If the pulse is long enough, the electrons will eventually be expelled from the channel by the radial light pressure. This would also produce a radially dependent index of refraction and thus can act to further guide the laser pulse, in a process called electron cavitation. Alternatively, a density-gradient channel could be preformed in order to extend the laser propagation distance, by use of another earlier pulse.
Collimated beam of MeV electrons
We have recently demonstrated that a laser wake field can produce a collimated beam of millionelectronvolt (MeV) electrons and that the laser may have been guided by relativistic self-focusing and electron cavitation.3 The experiment is remarkably simple. A high-power laser is focused into a molecular beam produced by a gas jet. The laser used in the experiment has a pulse duration of t = 400 fs and an energy up to 10 J, corresponding to a peak power of 25 TW. When focused in vacuum with an f/5 off-axis parabolic mirror, the laser can reach an intensity of up to 1019 W/cm2.
In order to create the plasma, the gas from a pulsed valve is tunnel-ionized by the laser pulse itself, reaching a peak electron density on-axis of 1019 cm-3.
In this case, the laser pulse width is much greater than a plasma period:
t >> 2/λwp, where wp is the plasma frequency. This condition is usually described as the self-modulated laser wake field because, in this case, the laser pulse becomes modulated by the plasma wave with a periodicity of a plasma period.
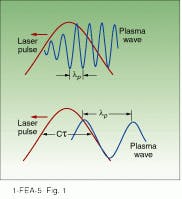
Basically, the density rarefactions of the plasma wave modify the index of refraction periodically along the axis of the pulse, breaking the pulse into a sequence of shorter pulses (see Fig. 1, top). It is related to the stimulated Raman scattering instability. With this scheme, greater than 108 electrons were observed to be accelerated. The figure at the top of this page shows the intensity profile of the laser at its best focus and the electron beam at a distance 8 cm away. Note that the spatial distribution of the electron beam appears to map well to that of the laser at its focus where the electrons were accelerated. What is remarkable is that, simply by focusing a laser into a gas, electrons are accelerated to relativistic energies in a well-defined beam.There is also some evidence that relativistic self-focusing may have produced a laser intensity on-axis of greater than 1020 W/cm2. The beam of electrons appears only at a laser power that matches the theoretically predicted relativistic self-focusing threshold. In another experiment with similar parameters, the electron energy distribution was measured to be flat, with a peak energy greater than 30 MeV.4 The maximum electron energy is limited by the detuning that occurs due to the difference between the electron velocity and the plasma wave phase velocity. The maximum predicted for the density of this experiment is approximately 100 MeV. However, higher maximum energy can be obtained with lower plasma density.
Resonantly driven plasma waves
In terms of future directions, better control of the plasma wave and higher final electron energies (up to GeV) can be achieved with a pump pulse with a pulsewidth t > 2λ/wp (see Fig. 1, bottom). The most efficient method to generate plasma waves with this property is called the resonant laser-plasma accelerator (RLPA),5 which uses a series of pump pulses with increasing spacing between them and decreasing pulsewidths to compensate for the change in resonance as the plasma wave grows and wp changes. In other words, rather than let the pulse break up into a series of pulses through the self-modulation instability, the optimal pulse train is instead created with the laser itself and then injected into the plasma.
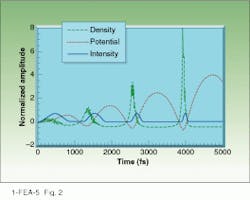
Because t > 2λ/wp, laser-plasma instabilities such as self-modulation (which grow on a timescale of t >> 2λ/wp) can be avoided. This is expected to provide better control and reproducibility of the plasma wave. Also, because for a given laser pulse width, this resonance condition implies a lower plasma density than in the self-modulated case, the laser beam and electron bunch can propagate in the plasma at closer to the speed of light, thus achieving higher final electron energies. Figure 2 shows the optimal pulse train determined by numerical simulation. The resulting plasma wave is an order of magnitude higher amplitude than would be obtained by use of a single pulse of the same energy with the same plasma density.Shorter electron pulses in a single bunch with lower electron energy spread can be achieved by more-controlled electron injection, which can be accomplished with a concept we call laser-injected laser accelerator (LILAC). Standard laser wake fields, such as those driven by the RLPA, have usually been considered primarily in the context of second-stage electron accelerators. In order to match the phase velocity of the wake, a trailing bunch of electrons must—prior to injection into the wake—be generated and preaccelerated to relativistic velocities by conventional means, such as with a medical linac. (The accelerated electrons in the self-modulated wake field experiment described above may have been preaccelerated by another slower-phase velocity plasma wave, which is difficult to control.) However, if a controlled means for injection of electrons could be found, then wake fields could be used to advantage in this first acceleration stage as well.
The low-field gradient (< 6.5 MeV/m) of a conventional first-stage accelerator prolongs the time during which beam emittance can grow before the beam becomes relativistic; after this point, self-generated magnetic fields can balance the effects of space charge. Even with state-of-the-art electron guns, the pulsewidth of the electron bunch can be considerably longer than the plasma wave period of a second-stage laser-plasma accelerator. It will thus fill multiple acceleration buckets uniformly in phase space, resulting in a large energy spread. Also, electron-beam positioning and focusing with micron accuracy and femtosecond synchronization between the electron beam and the plasma wave acceleration phase are difficult to achieve in order to overlap in both space and time. The lilac may serve to mitigate these problems.
Novel injection concepts
We have proposed two unconventional ways to inject electrons, both using a second laser pulse: either to dephase plasma wave electrons or create new ones by further ionizing the medium.7 The basic idea of the former scheme is that if a variably delayed injection pulse—propagating in the direction perpendicular to the propagation direction of a wake-field plasma wave, which is driven by a separate pump pulse—is brought to a focus at the correct point in space and time, then it can change the trajectories of the background electrons (oscillating in the plasma wave) such that they can become accelerated and trapped by the plasma wave (see Fig. 4). It is the ponderomotive force due to the transverse field gradient of the injection pulse that gives rise to the change in phase of the background electrons. In the latter concept, the second pulse is collinear with the first pulse but of higher peak intensity, thus creating new electrons from a higher ionization stage. Because these electrons are born in an intense field, they can be accelerated by the second laser pulse and become trapped in the plasma wave created by the first pulse, just as in the first scheme.By employing optical techniques and plasma waves for the generation as well as the acceleration of electrons, phase synchronization and spatial overlap are made much easier. Beam emittance can be improved by having a much higher field gradient in the first acceleration stage. The device can either be used by itself as a stand-alone accelerator system or as an injector for high-energy accelerators. The accelerated electrons can also be used to produce tunable short-wavelength radiation—either Bremstrahlung radiation from striking an anode as in an x-ray tube or synchrotron radiation when they are passed through undulator magnets as in conventional synchrotron light sources or free-electron lasers.
Applications
The electrons accelerated by lasers and the high-energy photons into which they can be converted have numerous industrial, medical, and scientific applications. Besides opening a new route to the next generation of TeV (teraelectronvolt) linear accelerators, laser accelerators can also produce single ultrashort duration (femtosecond) electron pulses—without the need for pulse selection or beam compression—making them ideally suited for studies of ultrafast dynamics. For instance, electron or x-ray diffraction could now be time-resolved on the femtosecond timescale. Synchrotron sources, in contrast, have durations that are at best 30 ps long.
And, because the electrons or x-rays are absolutely synchronized to the laser that produced them, they have the additional advantage of being capable of being used for jitter-free pump-probe measurements of photoinduced processes. Specific examples of other ultrafast-laser applications include pulsed radiology, material structural dynamics such as melting, temporal backlighting of dense plasmas, imaging of live biological cells, time-resolved absorption spectroscopy of either quantum controlled photo-initiated chemical reactions or transient energy states of laser-ablated materials (for material processing and thin-film deposition), photosynthesis dynamics, photoelectron spectroscopy (for condensed-matter surface studies), inner shell atomic ionization, and nonlinear optics with x-rays.
These new sources should be more readily available to a wider research community than synchrotron sources. In the next few years, we might expect to see an explosion of advances in, and applications of, laser accelerators almost rivaling the growth of applications immediately following the invention of the laser itself.
ACKNOWLEDGMENTS
This work would not have been possible without the efforts of a team of dedicated investigators: Szu-Yuan Chen, Evan Dodd, Joon-Koo Kim, Anatoly Maksimchuk, Torsten Neubert, and Robert Wagner. And without the invention of the CPA technique by Gerard Mourou and his coworkers, laser accelerators could only be imagined.
REFERENCES
1. P. Maine et al., IEEE J. Quantum Electron. 24, 398 (1988); G. Mourou and D. Umstadter, Phys. Fluids B 4, 2315 (1992).
2. T. Tajima and J. M. Dawson, Phys. Rev. Lett. 43, 267 (1979).
3. R. Wagner et al., "MeV-electron acceleration by the combined action of low- and high-phase-velocity plasma waves parametrically driven by a high-intensity laser," 37th Annual Meeting of the American Physical Society, Division of Plasma Physics, Louisville, KY, (Nov. 1995).
4. A. Modena et al., Nature 377, 606 (1995).
5. D. Umstadter, E. Esarey, and J. Kim, Phys. Rev. Lett. 72, 1224 (1994); D. Umstadter et al., Phys. Rev. E, 3484 (1995).
6. A. M. Weiner, J. P. Heritage, and E. M. Kirschner, J. Opt. Soc. Am. B 5, 1563 (1988).
7. E. Dodd, J. K. Kim, and D. Umstadter, "An ultrashort-pulse relativistic electron gun accelerator," 37th Annual Meeting of the American Physical Society, Division of Plasma Physics, Louisville, KY, (Nov. 1995).