Simulation software tackles design of laser resonators

Konrad Altmann

FIGURE 1. Graphical user interface can be used like an optical workbench on the PC and therefore permits intuitive design of laser resonators. Click here to enlarge image

Laser engineers are generally not inclined to use simulation software for optimizing resonator design for a number of reasons previously described in this publication (see Laser Focus World, Jan. 1998, p. 207). One of the main reasons historically has been that no software tool was available to rigorously analyze the complicated, multiphysics interaction of thermal and optical effects in solid-state lasers. Without this capability even highly sophisticated optics codes fail to predict the correct behavior of these lasers.

To fill this gap, more-recently developed software combines several simulation tools to provide rapid analysis of the complex interactions in a resonator design. For instance, the LASCAD software package developed by Micro Systems Design GmbH (MSD; Munich, Germany) includes finite element code for thermal and structural analysis, a Gaussian ABCD matrix algorithm, and wave-optics code based on the beam-propagation method with the additional capability of numerical eigenmodes analysis (see Fig. 1).

Laser engineering tool

The three-dimensional, nonlinear interaction of thermal and optical fields, commonly known as thermal lensing, is one of the key problems that designers of solid-state lasers are confronted with (see Laser Focus World, Dec. 1998, p. 129). This effect is of growing importance due to the tendency to miniaturize laser systems and simultaneously to increase their output power, causing the interaction of strong fields in very small volumes. Thermal lensing strongly depends on material parameters, resonator geometry, pump-beam distribution, and cooling layout. It interferes with gain guiding and other effects that control beam quality and efficiency in a complicated manner. Numerical simulation plays an important role in allowing laser engineers to preview and compensate for these effects prior to committing hardware to a design concept.

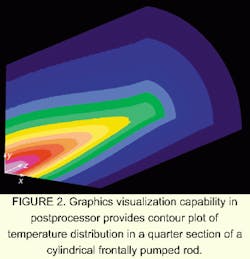
Finite element analysis (FEA) is used to compute temperature distribution, deformation, and stress or fracture mechanics in laser crystals and to analyze the effect of different cooling layouts. Though frequently applied in other engineering disciplines, FEA is not very well known in laser technology. So predesigned finite element models with adjustable parameters, such as dimensions and FEA mesh, are provided to assist laser engineers with common configurations, such as frontally pumped rods and blocks. Either super-Gaussian functions or numerical input can also be used to model pump-light distribution. A postprocessor with sophisticated graphics visualization capability can enable display and editing of FEA results (see Fig. 2). A finite-element preprocessor can also generate models for special configurations, such as composite laser materials with undoped regions. Models to fit many design needs may also be found in growing vendor library resources or can often be custom developed by the software vendor.

When FEA results are used with the ABCD matrix code in the LASCAD system, the temperature distribution multiplied by the temperature dependence of the refractive index is fitted parabolically at right angles to the optical axis using the finite element mesh subdivisions. In the same way, a fit of the deformed end faces of the crystal is carried through. For many configurations, frontally pumped rods, for example, this approximation delivers reliable results for the laser mode. Results of the ABCD matrix code, the fundamental mode spot size, and the phase front curvature along the resonator axis can be displayed, as well as higher-order Hermite-Gaussian polynomials. The ABCD matrix code completely takes into account astigmatism, and computations are carried through in two planes perpendicular to the resonator axis simultaneously.

FIGURE 2. Graphics visualization capability in postprocessor provides contour plot of temperature distribution in a quarter section of a cylindrical frontally pumped rod. Click here to enlarge image

Parabolic approximation and ABCD matrix code are not always sufficient, however. And in these cases FEA results can be alternatively used as input for a wave optics code. The wave optics code provides full 3-D simulation of the interaction of a propagating wavefront with the hot, thermally deformed crystal, without using parabolic approximation. For this purpose the code propagates the wavefront in small steps through the crystal, taking into account the transversal distribution of the refractive index, as well as the deformed front facets of the crystal, as obtained from FEA. A series of round-trips through the resonator is computed, which finally converges to the fundamental or to a superposition of higher order transversal modes. In addition, the wave optics code takes into account diffraction effects due to light stops and the finite transversal extension of mirrors. Mirrors with arbitrary reflectivity variations in radial direction can also be considered.

Another important capability of wave optics code is the simulation of misalignment influences that cannot be carried through in a satisfactory manner with the ABCD matrix code. For evaluation purposes, the wave optics code presents intensity and phase distribution at the outcoupling mirror, and thus provides important information about beam quality. This information may then be compared directly with physical wavefront measurements after the resonator is constructed. In addition, the wave optics code is capable of numerically computing the spectrum of resonator eigenvalues and also the shape of the transverse eigenmodes (see Fig. 3).1

In addition to the computation of resonator modes, the ABCD matrix code can also propagate the beam outside the resonator through an external optical system. This capability is currently implemented in LASCAD for the wave optics code. Gain distribution in LASCAD is currently taken into account on the basis of an overall power balance using the total loss of the resonator.

Development and evaluation

To verify the results of LASCAD simulation, MSD is cooperating with German laser companies and universities in a research project supported by the Bavarian Research Foundation (BRF; Bayerische Forschungsstiftung, Munich, Germany). One of these companies, LASOS GmbH (Jena, Germany), is simulating a microlaser with frontally pumped rod and has achieved good agreement with measurements. Richard Wallenstein's laser group at the University of Kaiserslautern (Germany) performs analysis and optimization of composite crystals in diode-pumped high-power picosecond lasers and amplifiers. A detailed series of measurements was carried out, which have determined the laser parameters carefully and verified the simulation results. The laser division of Daimler-Chrysler AG (Munich, Germany) which has cooperated with MSD in other research projects for four years, has achieved good results from incorporating simulation software into its laser systems. LDT Laser-Display-Technology GmbH (Gera, Germany) simulates long, folded resonators for second-harmonic generation.

Click here to enlarge image

FIGURE 3. Hermite-Gaussian mode TEM₂₂ was obtained by numerical eigenmodes analysis.

To facilitate comparison of simulated models with measurements of physical systems, MSD and WaveFront Sciences (Albuquerque, NM) are collaborating to allow easy translation of data between LASCAD and WaveFront Sciences' Complete Light Analysis System (CLAS-2D).