The nonlinear response
When the intensity of light in a transparent dielectric exceeds a certain threshold, the beam is no longer simply transmitted with its wavelength unchanged. A wide variety of phenomena create new beams with different wavelengths, and can reveal the strange quantum nature of light in ways that have no parallel in our everyday world. Nonlinear optics (NLO) is the science that describes the interaction of intense light with matter.
Nonlinear optical phenomena do not always require high-power lasers. The first NLO effects were discovered more than a century ago when high-voltage direct-current fields were applied to transparent materials. More recently, unwanted NLO effects have plagued optical networks, brought about by micron-sized fiber cores constraining beams at only milliwatt levels.
Constraining low-power beams in miniaturized crystals and thin films can also produce desirable NLO effects. Moreover a new class of nonlinear materials based on organic compounds may enable full-scale NLO effects using inexpensive milliwatt-sized lasers. Nevertheless, most NLO effects rely on intensities that can only be produced by high-power lasers.
The underlying physics
When light enters a dielectric, it is absorbed by the electrons surrounding the molecules, causing them to oscillate. The displacement of the electrons gives rise to polarization. The dynamics of the polarization itself then produces the light transmitted through the material.
This polarization is proportional to the electric field of the incident beam, P ∝ χE where χ is the susceptibility. This complex quantity contains all of the information about the optical properties of the material—its index of refraction (the real part), and its absorption (the imaginary part).
Just as when a mass on a spring is stretched past a certain point, an electron driven far from position no longer oscillates in a simple harmonic way. At sufficient intensities, the force of the light wave begins to match the force restoring the electron to its equilibrium position.
The fields that bind an electron to its molecule are generally in the range of 106 to 109 V/mm. The electric fields of conventional light do not exceed 102 V/mm, causing only small displacements. A laser, however, can produce an electric field on the same order as the molecular binding strength. The polarization of the material then oscillates at frequencies not present in the incident light, thus generating new wavelengths (see Fig. 1).The underlying math
The theory of nonlinear oscillations assumes that the response of the system depends on powers of the driving force. With the driving electric field expanded as a power series, the polarization response appears as
P µ χ(1)E + χ(2)E2 + χ(3)E3 + U ... [1]
The light field is a harmonic function, such as Ecos(wt). Trigonometric identities allow a power of a harmonic function, like cos2(wt), to be converted to terms like cos(2wt). The nonlinear terms in the polarization can then be grouped as
P µU+ χ(2)E2cos(2ωt) + χ(3)E3cos(3ωt) +U ... [2]
This is a simplified form of the fundamental equation of NLO. The electron cloud oscillates not only at the same frequency as the applied field, but also at multiples of that frequency. Although the dependence on E grows for each term, each succeeding susceptibility coefficient becomes very much smaller. In linear optics, higher-order terms are too weak to produce new wavelengths.
First seen here
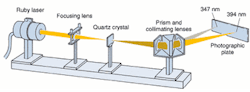
Beyond a threshold intensity, which depends strongly on the molecular structure of the dielectric, second-order effects start to appear. The quintessential NLO effect is second-harmonic generation (SHG), discovered in 1961 soon after the invention of the laser (see Fig. 2). It was immediately realized that SHG was a means to produce coherent light at shorter wavelengths.But success took decades to achieve. One of the limitations was the need to find materials that had refractive indices that could be manipulated to direct both the doubled and the fundamental beams. Converting two photons to one photon with twice the frequency must satisfy the conservation of momentum as well as energy.
This requirement translates into the need to "phase match" the doubled beam with the fundamental. In effect, the two beams must remain together in the material. The refractive index of the crystal must match for the two widely separated wavelengths. When this condition is satisfied, it maximizes the length of nonlinear interaction in the crystal. (Phase-matching will be discussed in detail in a future article in this series.)
An elastic theory
When more than one intense beam illuminates an optic, the polarization must account for the possibility that the beams have different wavelengths. In addition to terms like E2cos(2ωt), Equation 2 then includes terms for two beams, such as E1cos(ω1t) E2cos(ω2t), and so on for higher orders. New wavelengths can now be produced with frequencies other than multiples of the fundamental.
Setting one "beam" to have a frequency of zero, for example, represents an applied dc voltage. The application of a high voltage to a crystal such as KDP induces a birefringence that can be used for instance to control intense laser beams. This second-order change in the refractive index is the Pockels' effect, first discovered in the 19th century.
Third-order effects include third-harmonic generation, four-wave mixing and its many variations, two-photon absorption, stimulated scattering, self-focusing and other self-induced refractive effects. Higher-order effects in practice are limited to the laboratory generation of harmonics, although fourth-harmonic generation has found its way into some commercial products.
The list above is not exhaustive—for instance, a beam can produce a DC field in a dielectric proportional to the intensity of the light, which is a second order effect; another third order effect induces SHG in crystals that otherwise will not support it. Some effects are referred to by different names depending on the application.
The Kerr effect refers to third-order effects where the refractive index is altered by the incident beam. The earliest reference is to the birefringence induced by the square of an applied DC field (two "beams" of zero frequency). This illustrates how the formalism of Equation 2 can be manipulated to describe many physical cases.
Self-focusing is a third-order effect that increases the refractive index of the material as the intensity is increased, causing the beam to reduce in diameter. This results in a still greater change to the refractive index and more focusing. This runaway effect is a limitation in some systems that use high peak-power pulsed lasers.
This same third-order term also produces self-phase modulation. As an intense pulse propagates through the material, the leading edge experiences an increasing index of refraction. This causes a delay in the Fourier components of the pulse, producing a "red-shifted" leading edge. Conversely, the trailing edge is "blue-shifted," thus broadening the spectrum of the pulse. Compensating for such effects is critical in the design of ultrafast systems.
Inelastic scattering
This theory of nonlinear optics is "semi-classical"—the behavior of light is described by Maxwell's equation, while quantum mechanics is used to account for the energy levels of the dielectric. The dielectric, in effect, acts as a catalyst in the exchange of energy between light beams. However this semi-classical picture cannot describe all NLO phenomena.
The electrons in the material have a variety of cross sections for scattering the incident light. Rayleigh scattering of sunlight in the atmosphere, for instance, is what makes the sky blue. When the intensity of the beam being scattered exceeds a threshold, the character of the scattered light begins to change. It exhibits coherence.
This is called "stimulated scattering," and its theory goes beyond the semi-classical treatment. The creation of new photons using modes of molecular energy requires a fully quantum explanation. The most important examples are stimulated Raman scattering (SRS) and stimulated Brillouin scattering (SBS).
In SRS light loses or acquires energy from molecular levels, then re-emits the light at a different wavelength when the molecules return to their ground state. In SBS, light can create high-frequency acoustic waves in a lattice, which then scatters the light to different wavelengths.
While theoretically more complex, stimulated scattering in practice can be relatively simple. Because the material contributes to the exchange of energy and momentum, stimulated scattering does not need to be phase-matched. Moreover, most stimulated scattering processes do not require a crystalline host.
This is both a blessing and a curse. Variations of SRS are conveniently used in the spectroscopic analysis of fluids (see photo at top of this page). On the other hand, while the nonlinear coefficients of fiberoptic cable are extremely low, nevertheless the tight confinement of the beam combined with the long propagation distances make SBS a major source of noise in high-speed networks.
New dimensions needed
So far, the discussion has only considered the one-dimensional case, of polarized electrons oscillating in the direction of the light wave. However when an electron in the dielectric lattice is displaced from equilibrium, it will experience not only a restoring force, but also forces from the neighboring molecules. A field applied in the x direction may result in an electron moving in the y and z directions as well.
The strength and direction of these forces depend very much on the structure of the crystal. To describe the generation of new light in different directions, the susceptibility must be a tensor quantity. A basic understanding of tensors is found to be essential in specifying or developing dielectric materials for NLO applications.
Next month's article in this series will discuss the theory and practice of nonlinear optical materials.