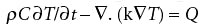Internal heating is a key issue for vertical-cavity surface-emitting lasers (VCSELs), which generally have higher thermal impedance than edge-emitters. VCSELs are fairly efficient for optical devices, running at roughly 25% efficiency, in contrast to LEDs, which are typically 2% to 3% efficient. Even so, for a 40-mW input they produce 10 mW in optical power with the remaining energy dissipated as heat.
How easily a laser dissipates heat varies with its physical design. VCSELs are cubes whereas edge-emitters are fabricated as long, flat chips, so the edge-emitter active area (where heat is generated) has much broader contact with the heat-removing substrate. Another factor is that the mirrors on VCSELs consist of epitaxial films a few nanometers thick on the top and bottom, giving them a higher thermal resistance than an edge-emitter in which the mirrors reside on the edges of the bar. This configuration again makes it easier for heat to escape through the edge-emitter substrate.
Heat buildup in the active region of a laser can reduce device efficiency along with reliability and operating lifetime. Further, VCSELs are narrowband devices, in this case generating energy at 850 nm. As a VCSEL heats up, the length of the optical cavity changes and the wavelength shifts at a rate of roughly 0.6 angstrom/°C.
A study was envisioned at Honeywell Laboratories to estimate the total temperature increase around the VCSEL active region as a function of drive current. One goal of the study was to determine the effects of going from a die size of 350 µm on an edge to 220 µm. This reduction would allow more devices per wafer, leading to lower manufacturing costs. However, we first had to determine the effects of this smaller size on internal heating and thus reliability, especially because of a reduced cross section through which heat could escape, and thus a higher thermal resistance.
The modeling process
As a specific goal we chose to examine the temperature distribution inside a 350-µm VCSEL die made of gallium arsenide (GaAs) with a gold bonding pad on top. Each die was bonded at its base to a ceramic pedestal with a silver epoxy.
In the geometry of a VCSEL cube drawn with the graphics editor, the gold pad on the top of the die in the center is the aperture through which the light leaves the device (left). In mesh created for the geometry, a tighter mesh focuses on areas of high interest while coarser mesh helps conserve memory space and time (center). Temperature distribution inside the VCSEL die with drive current at 40 mA shows that most heat escapes through the bottom (right).
The first step in our approach to this problem using Femlab (COMSOL; Burlington, MA) modeling software was to select an application mode based on the appropriate differential equation—in this case, a three-dimensional model of heat transfer by conduction. The next step was to draw the actual model geometry using computer-aided-design (CAD) tools (see Fig. 1a).
Following the rendering of geometry was the definition of boundary conditions based on several assumptions: that the VCSEL was the only heat source; that all surfaces in direct contact with air would lose heat at a rate governed by Newton's Law of Cooling; and that the coefficient of heat transfer would be h = 0.1 W/m2 – K. Conditions at the bottom face were also important because in a real application the die attaches there to the physical package and provides a path for heat to flow. This model assumed that the package was attached to a large heat sink that could handle as much heat as the device could produce, so it set the base of the VCSEL die at a constant ambient temperature.
To help in setting these boundary values and also in verifying the model's validity, we measured the actual temperature on the top face and sides of the VCSEL (coated with India ink to make them highly emissive) using an infrared camera. Then, we bonded a miniature thermocouple on the device base and found that results from the camera and thermocouple agreed quite well, which made us confident that the camera-based temperatures would be valid on all device surfaces.
The next step was to set the coefficients for the underlying heat equation:
null
The model considered only the steady-state case, so it ignored the first term and dealt with three different subdomains—the GaAs, the gold, and the VCSEL itself—each with its own thermal conductivity.
With the model set up, the software generated a mesh for the entire geometry and we set up the model so that a tighter mesh resulted in areas of highest interest, in this case, the gold pad on the top surface, only 1.5 µm thick. The remainder of the model worked with a relatively coarse mesh to help conserve memory space and reduce the solution time (see Fig. 1, center).
The solution generated a graphical plot of the results showing the temperature distribution inside the VCSEL die and reading out the temperature at a specific point that we could also read with the camera, helping to verify the model's validity (see Fig. 1, right).
For each experiment involving a different geometry or material formulation, we ran about 20 models, each with a different drive current. We found that the difference in measured values of temperature and those obtained from modeling was ±0.2°C. Each model, run on Femlab 2.0, took roughly two minutes on a Pentium II machine with 128 MBytes of RAM; with the latest release, Femlab 3.0a, this same model takes roughly 10 seconds.
The experiments substantiated the original hypothesis that most of the heat generated by the drive current goes through the substrate and that only a small amount is radiated into the ambient air directly from the device. With this model we were able to test and verify new design concepts such as alternative doping profiles, aperture diameters, and levels of drive current. Further studies showed that various types of solder and thermoconductive ceramic bases had little effect on the final results. We concluded that we would be able to go to a smaller die size without any significant negative effect on device reliability.
J. ALLEN COX is a senior research fellow at Honeywell Laboratories, 12001 Highway 55, Plymouth, MN 55441; e-mail [email protected].
Moving into new areas
With the acquisition of Honeywell's VCSEL Optical Products business by Finisar (Sunnyvale, CA) last spring, our modeling projects have shifted to new areas. One of those areas is the examination of the optical scattering from blood cells as a means of identifying blood types. This problem becomes complicated to model because of complex geometries. A typical white blood cell is 10 µm in diameter and very irregularly shaped. Further, the nucleus and organelles are also irregularly shaped with little symmetry. Thus, there is no closed-form model to predict the optical scattering.
A rough approximation of this scattering can be obtained using a Mie model, which although it encompasses all possible ratios of diameter to wavelength, deals only with spherical objects and assumes a homogeneous, isotropic and optically linear material. To get better results, others have tried to work with a FDTD (finite-difference time-domain) scattering model, but we believe that the FEA (finite element analysis) method should also work well based on previous experience with finite element modeling of diffractive optics. We are starting out by making some simplifying assumptions about the geometry, given the knowledge that such a cell is roughly elliptical and we also know the general shape of the nucleus. Further, there is no Femlab application mode predefined for optical scattering, but the package allows users to enter their own partial differential equations directly.