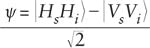Novel Fiber-Optic Applications: Microstructured fiber entangles light for quantum information processing
JINGYUN FAN and ALAN MIGDALL
Quantum information science provides unprecedented improvements in information processing and is moving out of the laboratory. Large-scale systems consisting of hundreds of quantum bits (qubits) are being built to conduct quantum computational tasks. With communication security dictated by the laws of quantum mechanics (rather than simply relying on the fact that cracking current communications systems is a very difficult and time-consuming task), several secure quantum networks are being built in China, Japan, Europe, and the United States.
Besides investment from government, the private sector is also expressing strong interest in secure quantum communications that transport signals thousands of miles across single-mode optical fiber (SMF) to deliver secure information between users.
Compared to atomic qubits, photonic qubits possess unique advantages. Resistant to many decoherence mechanisms and acting as the fastest information carriers, photonic qubits are inarguably the choice to carry information to a remote location, ending with detection or conversion into atomic-stationary qubits.
Proof-of-principle experiments on quantum computing protocols produce photonic qubits mainly via nonlinear wave-mixing methods. The primary workhorse is spontaneous parametric downconversion (SPDC), which takes a single photon from the driving laser field and creates a pair of daughter photons. SPDC was initially demonstrated in bulk crystals, but cavity and waveguide-based SPDC processes are of recent interest. One distinct feature in these processes relative to bulk SPDC is that the produced pair of photons exists in one or few spatial optical modes, which is essential to many quantum information application tasks.
Following the maturing of SPDC, it was a natural progression to generate photon pairs via four-wave mixing (FWM) in single-mode optical fiber, with the advantage that preparation and consumption of photonic qubits for information processing takes place seamlessly within the existing optical fiber communication network. Though it may appear that FWM photon-pair generation is less efficient than SPDC (because FWM is third order, while SPDC is a second-order nonlinear optical process), the brightness and quality of photonic qubits created via FWM in optical fiber is competitive with SPDC.
Today, FWM is a common tool implemented in many quantum optics and quantum information technology laboratories as an alternative to SPDC. And at NIST, we have explored how microstructured fiber can be specifically engineered to take advantage of these FWM processes to generate high-purity entangled photons critical to cutting-edge secure quantum encryption and computing applications.1
Microstructured fiber four-wave mixing
In glass-based single-mode optical fiber, the inversion symmetry of glass makes the third-order nonlinear optical interaction the leading process. Thus, this otherwise weak nonlinear process can become noticeable thanks to the single-mode guiding geometry and the phase-matching mechanism that can turn a few hundred meters of optical fiber into a coherent source of photon pairs.
Nonlinear fiber optics has been a familiar subject to many of us. Raman scattering and FWM can be detrimental in optical communication because both mechanisms take energy away from the optical signal and create photons at new wavelengths. However, FWM can also be used to our advantage.
It takes two photons from the driving laser field(s) (ωp) to create a pair of signal (ωs, ωs greater than ωp, ω = ωs–ωp, sometimes referred to as anti-Stokes) and idler (ωi, ωi less than ωp, ω = ωp–ωI, sometimes referred to as Stokes) photons that are constrained by energy conservation, or ωs + ωi =2ωp. These pairs of photons can be used to create heralded single photons or photonic entanglement—at the desired wavelengths (by tuning the structure of the fiber—to suit a variety of quantum information application tasks.
Raman scattering couples photons to the phonon bath [n(Ω) = 1/(ehΩ/kBT)–1] of optical fiber and scatters photons from the driving field probabilistically into a continuous-noise photon background that is significant over a 40 THz spectral range and peaks around 13 THz higher than the driving laser frequency. Operating standard optical fiber at cryogenic temperatures can reduce the phonon population as a way of reducing the single-photon noise; however, microstructured fiber offers an elegant cryogenic-free solution.
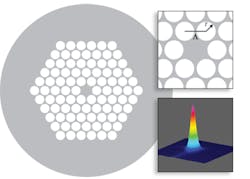
While microstructured fiber is also made of glass material, its core is suspended by a few silica membranes in the air like a spider centered on its web (see Fig. 1). The dramatic difference in the refractive index between core (ncore = 1.45) and cladding (nclad ~ 1) makes it possible to have small-core-mode diameters, for example, of 1 μm or less, while maintaining the single-mode guiding property for light ranging from the ultraviolet to the near-infrared region.
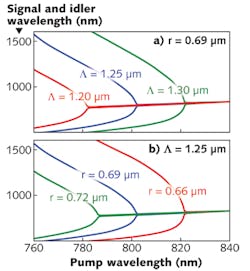
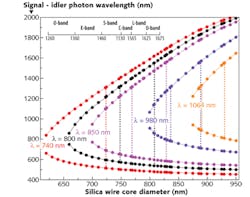
The reduction in the optical-mode area greatly enhances the optical nonlinearity, which is proportional to the intensity (I) of the light in the optical fiber. This allows the use of a few-tens-of-centimeters section of microstructured fiber to achieve a similar photon yield as a few hundred meters of standard single-mode optical fiber. And because the dispersion of microstructured fiber is sensitive to the air filling factor in the structure, the control of this critical property of dispersion can be achieved by physical dimensions rather than having to find a different material that is often not available. A small variation in this filling factor can displace the phase-matched FWM signals to very different wavelengths, with the signal and idler wavelengths being sensitive to small changes in the diameter and spacing of the holes in microstructured fiber (see Fig. 2).Experimental success
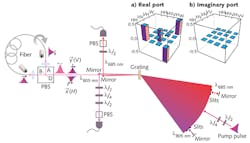
In practice, the pump wavelength is used to precisely tune the FWM process. A spectroscopic study of nonlinear processes in optical fiber shows that as the pump wavelength approaches the zero-dispersion wavelength of the microstructured fiber, the wavelength of FWM signals move further from the pump wavelength while the Raman signal peak is fixed relative to the pump frequency (see Fig. 3).In each FWM process, the lowest non-vacuum quantum state of FWM output may be approximated as Ψ~|HH), describing the quantum state of a pair of one-signal and one-idler photons. Correspondingly, the two two-photon states from the two FWM processes interfere coherently on the PBS, generating at its output polarization-entangled photon states as
With appropriate phase control, the Bell state
is created with measured fidelity of 99%. For this experiment, the microstructured fiber zero-dispersion wavelength is 745 +/-5 nm, nonlinearity = 70 W-1km-1, and length = 2 m. The pump wavelength was 740.7 nm with a repetition rate of 76 MHz and pump pulse duration of 10 ps. Signal and idler wavelengths are 689 and 800 nm, respectively, and the photon collection bandwidth is 0.9 nm. The experiment demonstrates that, through precise phase-matching engineering, one can produce photon pairs via FWM at the desired wavelengths with low single-photon Raman noise.
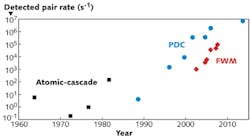
Microstructured fiber-based sources of photonic entanglement have progressed greatly in the past few years. The performance of such a source, measured by the two-photon spectral brightness and entanglement fidelity, is now approaching that of the best SPDC source (see Fig. 5). The flexibility in engineering the fiber gain, dispersion, and birefringence makes it possible to produce photonic entanglement over a wide spectral range in a single optical spatial mode, or to produce spectrally uncorrelated entanglement to meet a broad range of applications in quantum information technology.Improving microstructured-fiber entanglement
Despite progress in the development of microstructured-fiber-based photonic entanglement, several issues need to be addressed before these sources can enjoy widespread use.
First, the physical spider-web structure upon which nonlinear wave-mixing depends is subject to manufacturing defects and nonuniformity issues, and second, fiber dispersion varies noticeably for microstructured fibers, even for sections as short as a centimeter. This complicates the nonlinear interaction process, making it less efficient and broadening the spectrum of photons generated in the nonlinear wavelength conversion.
And finally, although the structure forces photons to propagate in a single-transverse optical spatial mode, the optical mode may exhibit poor rotational symmetry and vary significantly across fibers (or even from section to section in a single piece of fiber), thus requiring significant spatial-mode reshaping to remove the distinguishability that it imprints on the photons.
Micro-optical fibers with diameters less than 1 mm are made by heating and stretching a section of regular single-mode optical fiber and may be considered as a variation of microstructured fiber. An adiabatic transformation ensures that light propagates from the ordinary unmodified single-mode optical fiber on one end through a tapered section to the submicron fiber section and back out through another tapered section to the unmodified fiber with near-unity transmittance.
The small Gaussian-like mode profile of microfiber possesses rotation symmetry around the fiber axis and translation symmetry along the fiber length. Its effective optical nonlinearity is better than that of microstructured fiber and its optical dispersion can be tailored by precisely controlling the micro-optical fiber diameter (see Fig. 6).As opposed to microstructured fibers that can only be manufactured with special equipment, micro-optical fibers can be made in any lab with high-power lasers or hydrogen flames to heat the fiber. Looking to the future, microstructured fiber and its variations has great potential for groundbreaking quantum information applications.
REFERENCE
1. Jingyun Fan et al., IEEE J. Sel. Top. Quantum Electron. 15, 6, 1724–1732 (2009).
Jingyun Fan and Alan Migdall are physicists at the National Institute of Standards and Technology, 100 Bureau Dr., Gaithersburg, MD 20899. Fan and Migdall also belong to the Joint Quantum Institute of the University of Maryland, College Park, MD 20742; email: [email protected]; www.nist.gov.